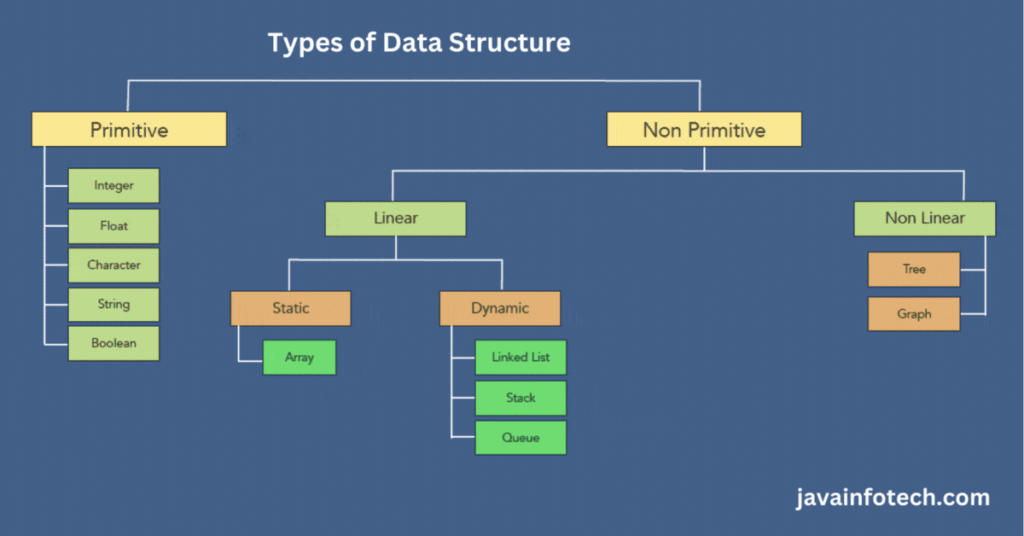
Types of Data structures are classified into two types: Primitive and Non-Primitive.
Primitive Type:
- Primitive data structures are the basic building blocks of any programming language. They include integers, floating-point numbers, characters, strings, and Booleans.
- Primitive data structures are typically stored in a contiguous block of memory and can be accessed directly using their memory address.
| DATA STRUCTURE | DESCRIPTION | EXAMPLE |
| INTEGER | Numbers with our decimal point | 1,2,3,4,5,300 |
| FLOAT | Numbers with decimal point | 2.5, 5.6, 4.8, 67.8 |
| CHARACTER | Single Character | A, B, C, F, D |
| STRING | Text | Hello, Data DSA |
| BOOLEAN | Logical values true or false | TRUE AND FALSE |
Non-Primitive:
- Non-primitive data structures are more complex than primitive data structures and are typically used to store collections of data.
- Examples of non-primitive data structures include arrays, linked lists, stacks, queues, trees, and graphs.
- Non-primitive data structures are typically stored in a non-contiguous block of memory and must be accessed using a pointer.
Primitive data structures are typically more efficient than non-primitive data structures because they are stored in a contiguous block of memory and can be accessed directly using their memory address. However, non-primitive data structures are more versatile than primitive data structures and can be used to store more complex collections of data.
Types of Algorithms:
- Simple recursive algorithms
- A Simple recursive algorithm calls itself with modified inputs until it reaches a base case, where the solution can be computed directly without further recursion.
- These algorithms are characterized by their straightforward implementation and reliance on the concept of recursion, making them easy to understand and implement for problems that exhibit repetitive or self-similar structures.
2. Divide and conquer algorithms
- Divide the problem into smaller subproblems of the same type, and solve these subproblems recursively.
- Combine the solutions to the subproblems into a solution to the original problem.
Examples: Quick sort and merge sort
3. Dynamic programming algorithms
- They work based on memorization.
- To find the best solution.
4. Greedy algorithms
- We take the best we can without worrying about future consequences.
- We hope that by choosing a local optimum solution at each step, we will end up at a global optimum solution.
5. Brute force algorithms
- It simply tries all possibilities until a satisfactory solution is found.
6. Randomized algorithms
- Use a random number at least once during the computation to make a decision.